Dimiter Prodanov
Exact path simulations for real and complex diffusions
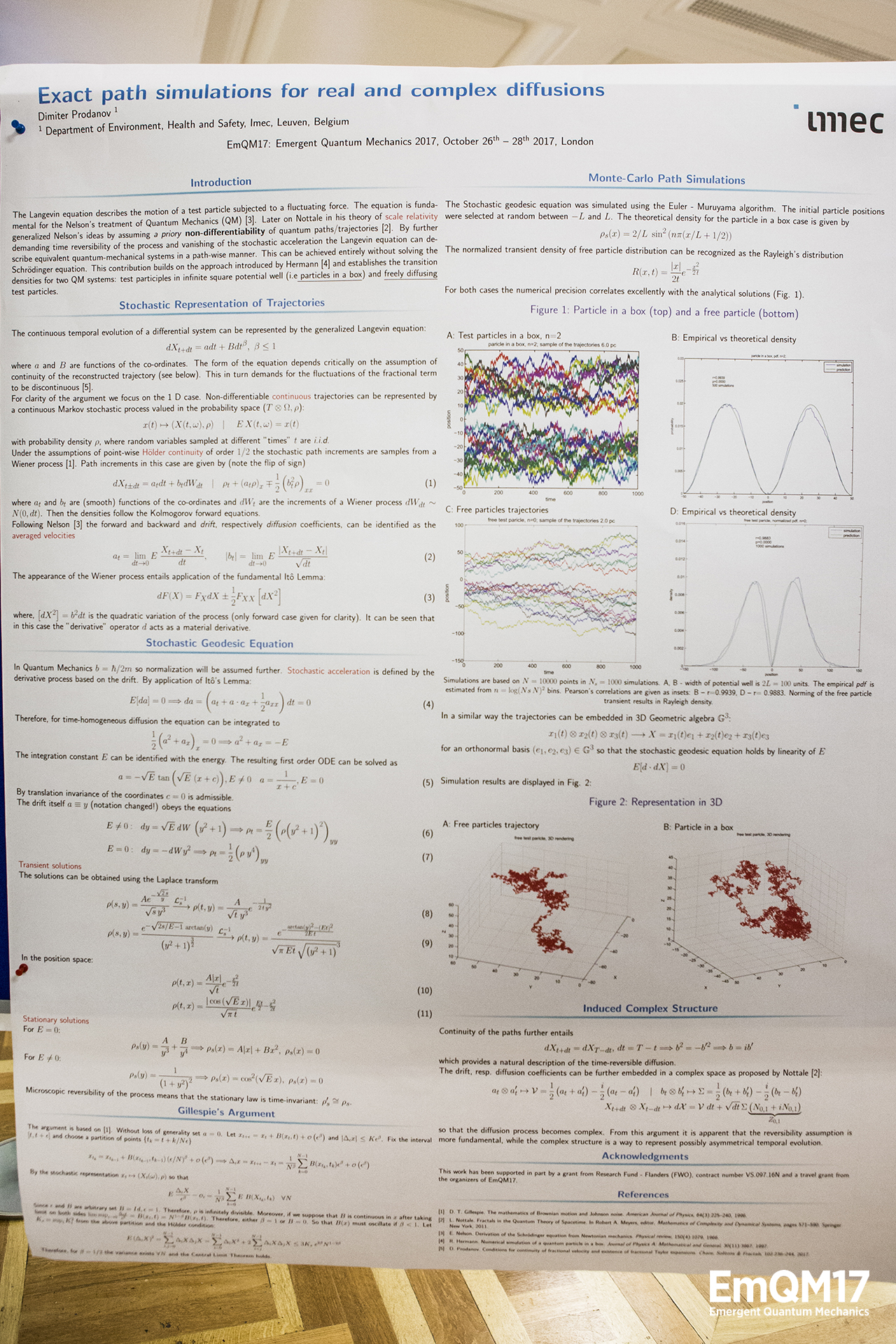
It is well known that the Langevin diffusion equation admits path-wise simulations by means the Gillespie algorithm. The properties of the equation depend critically on the assumption of continuity of the reconstructed function. This in turn demands for the fluctuations of the diffusion term to be discontinuous. Statistics of Monte-Carlo stochastic path simulations are equivalent to solutions of the Fokker-Plank equation of the probability density of the differential system. By further demanding time reversibility of the drift the Langevin equation can describe equivalent quantum-mechanical systems in a path-wise manner.
The use of the Langevin equation can be interpreted in Nelson’s stochastic quantum mechanics and Nottale’s scale relativity theory. Both theories admit complex structure of the driving process resulting, which provides a natural description of the time-reversible diffusion. This contribution studies simulation of free particles in infinite potential well. Achieved numerical precision correlates excellently (rho>99%) with the analytical solution using the Fokker-Plank equation. Exact formula for the process increments is demonstrated. Solutions are derived following the methods of moments, which achieves convergence in probability.